In last month’s blog we introduced sunspots. Most courses and books on astronomy then move on to the solar cycle, an approximately 11-year periodicity of solar activity, most clearly seen in the number and location of sunspots. However, we will take a digression to look at solar rotation. This is because to understand the nature and cause of the solar cycle we need an understanding of both the Sun’s rotation and its magnetic fields.
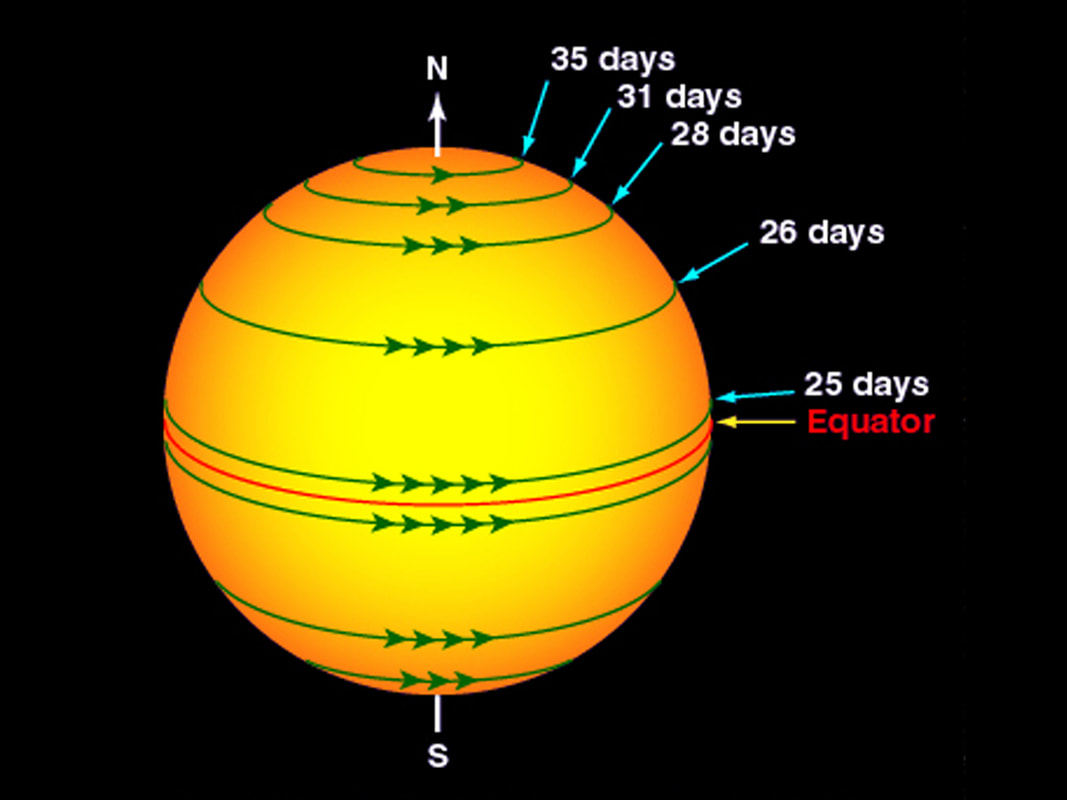
The idea of the Sun rotating on its axis had been proposed by several pre-telescopic era scientists. However, it was only when the telescope was invented and used that this could be confirmed by observations. It was probably Thomas Harriot (b.1560 d.1621), an English mathematician and astronomer who first observed sunspots in December of the year 1610. Early observations placed the rotation period of the Sun as from 25 to 28 days based on the apparent transit (moving across the face of the Sun) of the sunspots.
Le mois dernier nous avons parlé des taches solaires. La plupart des livres d’astronomie passent ensuite au cycle solaire (une période de 11 ans pendant laquelle l’activité du Soleil varie). Cette activité peut être vu dans le nombre de taches solaires. Maisd’abord nous allons parler de la rotation du Soleil parce qu’il faut compendre ce phénomène et les champs magnétiques du Soleilavant de comprendre la nature et la cause du cycle solaire.
Plusieurs scientifiques de l’époque pré-telescopique avaient proposé l’idée de la rotation solaire. Mais avant l’invention du télescope on ne pouvait pas la confirmer. C’est Thomas Harriot (1560-1621) un mathématicien et astronome anglais qui a été le premier observateur des taches solaires en decembre 1610. Les premiers observateurs supposent que la période de rotation du Soleil estentre 25 et 28 jours (à cause du transit des taches solaires devant le Soleil)
Carrington’s formula is what is known as an empirical formula, i.e. it is a formula based upon and derived from the observed data. Empirical formulæ are ‘best-fit’ relationships to the data available; they are not based upon theory or an understanding of the physical processes taking place. Empirical formulæ feature in many branches of science and often precede theoretical advances and/or laws based on known physics. For example, Kepler’s laws were derived empirically by Johannes Kepler. The ‘reason’ why they are such good matches to the observations was not explained until Newton’s work on gravity provided their basis.
Pre-twentieth and twenty-first century determinations of solar rotation were based upon sunspot transits. Nowadays, the array of techniques and methods also includes Doppler shift methods and helioseismology. The results we have today show a complex picture; as could perhaps be anticipated from what we know. The Sun, after all, is a huge sphere of hot plasma, with turbulent convection, different energy transport mechanisms, varying density, temperature and pressure gradients, and a very significant and complicated magnetic field.
The empirical equation for the observed latitudinal variation has evolved from Carrington’s formula and is now expressed in the form of a truncated sine (Fourier) series. Current best observational data for the Sun’s equatorial rotation period is 24.47days. Polar rotation period is around 35 days.
The rotation period we see from Earth is higher than the above times as the Earth is moving in orbit around the Sun in the same direction as the Sun rotates. This direction is known as retrograde motion.
If we were looking ‘down’ on the Sun’s North Pole we would see the Sun rotating anti-clockwise. All the major planets also orbit the Sun in retrograde (i.e. anti-clockwise) orbits. Rotation and revolution (orbiting) in the opposite, clockwise, direction is termed direct motion.
The Synodic period (which includes the Earth's orbital motion) of solar rotation, at the equator, is 26.24 days.
Sub-photospheric rotation
Today, we can see that the Sun does indeed have a differential rotation period depending upon the heliographic latitude. However, this is not consistent with depth (radial distance).
At the Tachocline (recall:- this is the zone where radiation dominance as an energy transfer mechanism gives way to convective processes) the differential rotation abruptly changes. The radiative zone and core appears to have a fixed, stable and consistent rotation; as would be exhibited by a solid sphere.
There is currently no comprehensive theory or physical explanation for the solar rotation.
More details on this, and the empirical equations modelling the observed photospheric rotation, are given within our textbook on the Sun.