As we have seen, with the exception of the Vulcanoids, asteroids are found in all regions of the solar system. However, many of the asteroids, particularly those outside the main belt, are on chaotic / unstable orbits. These asteroids often cross the orbits of the planets and as such, will on occasion come under the influences of gravitational perturbations (disruptions) from the major planets. Thus, their orbits can be changed and, from humanity’s perspective, these asteroids can become PHA (potentially hazardous asteroids) and a real risk to human kind.
On trouve les astéroïdes (sauf les vulcanoïdes) dans toutes les régions du système solaire. Cependant beaucoup de ces astéroïdes se trouvent sur des orbites instables. Ils traversent les orbites des planètes, et de temps en temps ils subissent les influences des planètes majeures. Ainsi leurs orbites peuvent changer et ils peuvent devenir des d'astéroïdes potentiellement dangereux.
Since the time the Earth formed, and life evolved, at least one, and possibly three, mass-species-extinctions has been initiated by asteroidal impact on the Earth. (We will look at each of these in future blog articles).
However, whilst gravitation is the dominant perturbing and orbit-changing force, it is not the only disturbing force or effect. Over the next 3 monthly blogs, we will look at the ways in which asteroid orbits can change. These can be categorised into 2 types of mechanism, gravitational and non-gravitational forces. We look here now at gravitational forces.
Many asteroids are on orbits which take them close to the major planets. On near approaches, the orbit of the asteroid is changed. This can be modelled to a high degree of accuracy using Newtonian gravitation and in particular with a mathematical model known as the restricted 3-body problem. This is where the asteroid is considered of negligible mass compared to the Sun and the major planet, or in other words, the gravitational influence of the asteroid’s mass on the major planet and the Sun can be ignored. In the case of asteroids, the model is very appropriate and accurate; the mass of the most massive of all asteroids, Ceres, is less than one-millionth that of Jupiter and just one ten-thousandth that of the Earth.
Asteroids (and comets) are mostly very small and cannot be observed throughout their orbit. This begs the question that if orbits can be changed, how do we know that the object we’re observing today is the same object that we saw previously? This apparent conundrum was solved by the French astronomer and mathematician Felix Tisserand. (We took a brief look at the life of Tisserand in our blog of and our comprehensive biography publication on his life is available )
Tisserand determined the orbital invariant which remained constant before, throughout and after such a gravitational encounter. The Tisserand criterion, T(c), relates semi-major axis, orbital eccentricity and orbital inclination. Tisserand derived this invariant from the Jacobi integral, named after the German mathematician Carl Gustav Jacobi (b.1804 d.1851). The criterion is in effect a statement of the conservation of angular momentum and orbital energy.
Tisserand’s criterion gives a single (unique) number by which to refer to an asteroid (or comet). When a new or recovered asteroid is observed the first thing which astronomers do is to calculate its orbit and its Tisserand number. The Tisserand criteria used by the MPC (Minor planet centre) and NASA’s Jet Propulsion Laboratories (JPL) is a modified form of and their measure T(j) is parametrised against the semi-major axis of Jupiter.
The mathematics of the Tisserand criteria T(c) and T(j) and the ‘Kozai mechanism’ which is also seen in research papers, is provided within our publication “The Asteroids of the solar system”.
The gravitational interactions between masses is more generally known by the name ‘perturbation theory’. Gravitational systems involving more than two massive objects are very complex to solve, and only very specific cases of analytical (formula-based) solutions are available even in the case of the restricted (i.e. the third object is massless) problem. The Lagrangian points are specific cases, which we briefly encountered when we discussed the Trojan asteroids in our blog of July 2019 .
A branch of mathematics called numerical analysis is needed to solve the differential equations. This effectively allows a computer model to be produced to show the future (or past) motions of the bodies.
However, some general results from perturbation theory, the mathematical modelling of effects of a perturbing force, e.g. the gravitational force exerted by a major planet upon a heliocentric orbiting body, can be quantified.
An historically classic example of perturbation theory is the case of Mercury’s orbit. The influences of Venus and Jupiter, and to a lesser extent all the other major planets, can be seen to effect changes in the Mercurian orbital eccentricity (e) and the celestial longitude of the perihelion point. Over time, the changes to the eccentricity cancel/average out, but the change in longitude of the perihelion point is consistent and advances at a calculate-able rate.
The rate calculated, initially by Urbain LeVerrier, taking into account all the major planets (574 arc seconds per century) was slightly at odds with the observed behaviour (531 arc seconds), by 43 arc-seconds per century, and this led to the search for Vulcan (as we discussed in last month’s blog). This small but measurable difference was one of the pivotal demonstrations of Einstein’s theory of general relativity.
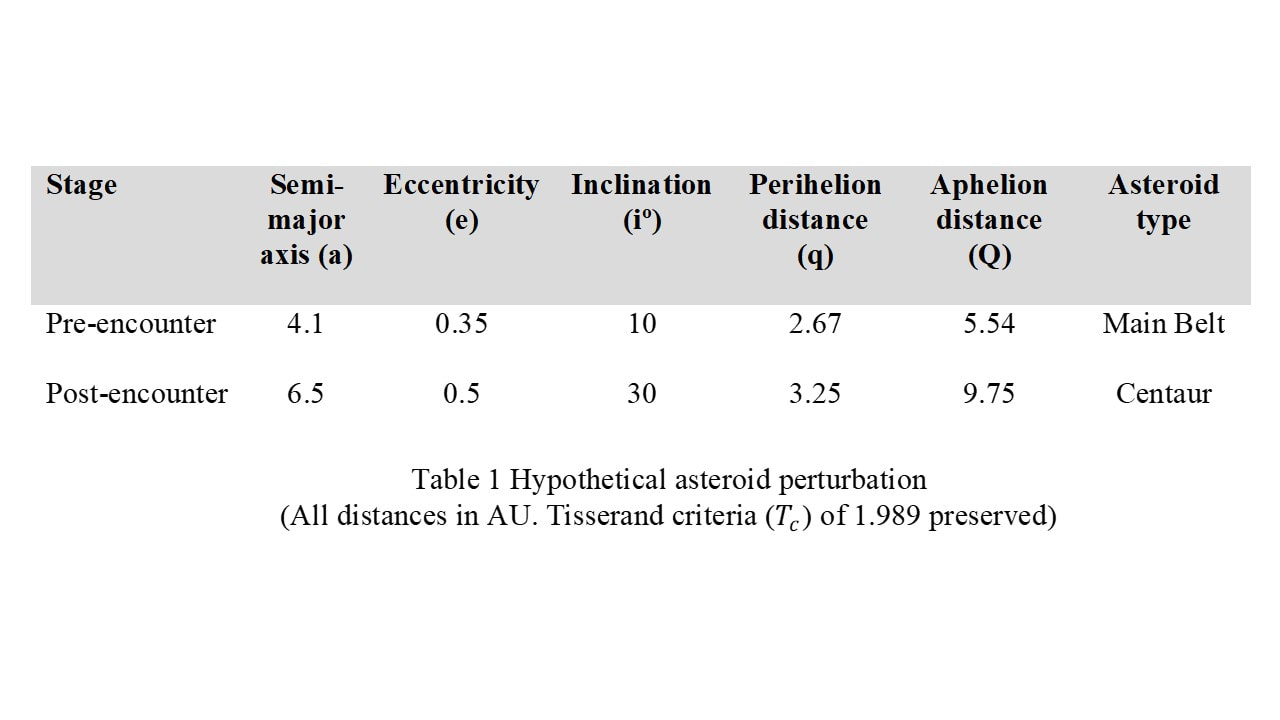
What both the Tisserand and Kozai mechanisms show is that whilst there are conservation principles (energy and angular momentum) acting, the changes to an asteroid’s specific orbit can be dramatic. The table below shows an example how a hypothetical asteroid’s orbit could change if, during its aphelion passage, it came close to Jupiter.
(All distances in AU. Tisserand criteria () of 1.989 preserved)
Dynamical modelling (by Observatoire Solaire) of the effects of Jupiter on asteroids has shown the possibility of a sparse second asteroid belt between the orbits of Jupiter and Saturn. The population of Centaurs will undoubtedly have some asteroids with this type of orbital migration history.
In our next regular blog we will take a look at the rather more complex and difficult to model, non-gravitational forces which affect asteroids.