In local news, we are pleased to say we have reprinted our book on the Limousin asteroid impact of the late Triassic and this (ISBN 978-1-9999044-1-8) is now available in paperback form from our bookstore.
We have now had this printed (perfect binding) in a very convenient A5 sized and at just £6.99 we would recommend this as an ideal read for anyone planning a holiday to France as well of those interested in asteroids and their interaction with the Earth.
Having just been passed through the Winter Solstice point in its orbit, the Earth passes through its perihelion point (the point at which it is closest to the Sun) on the 3rd of January 2018, at 05:35hrs UTC (06:35 CET); 3hrs 7mins and 2hrs 8mins before sunrise in Edinburgh and Paris respectively
Our blog this month looks at astronomical distances.
Merci à tous ceux qui ont aimé notre page facebook. Si vous aimez la page vous pourriez inviter vos amis à l'aimer. Notre dernier blog mensuel est maintenant disponible à link....
Après le solstice d'hiver (21.12.2017) la Terre va passer à son périhélie le 3 janvier 2018 à 05.35h UTC (06.35h CET) 3h 7min avant le lever du soleil à Edinburgh et 2h 8min avant le lever du soleil à Paris.
Nous sommes ravis d'annoncer que notre livre sur l'impact de l'asteroïde en Limousin a été republié. Le livre est maintenant disponible en format de poche (A5 parfaitement relié) dans le magasin sur notre site. (ISBN 978-1-9999044-1-8)
Ce mois nous allons considérer les distances astronomiques et vous donner un aperçu de l'echelle de l'Univers.
Le blog prochain sera publié samedi 27 janvier et nous allons considérer la question suivantes: si 98% de la masse baryonique dans l'univers est l'hydrogène atomique et l'helium l'on doit se demander pourquoi
ces éléments n'existent pas sur la Terre.
The ancient Greeks, and before them the Babylonian and Egyptian astronomers, knew that the stars were extremely distant from the Earth. They could detect no parallax (the effect whereby closer objects looked to be in slightly different positions against the background of more remote objects, when the closer objects are viewed from different positions) and they correctly deduced they were far more remote than either the Sun or any of the planets.
The sphere of stars was deemed to be fixed, unchanging and at an unmeasurably immense distance. The Sun, planets and the Earth’s moon however were known to be much closer and to be ‘dynamic’, moving against the background of the stars. Up to the time of the invention of the telescope (and particularly the publication by Galileo of his observations), the nature of the planets of the solar system was unknown. The etymology of the word ‘planet’ derives from the ancient Greek language (asters) plenetai – wandering (star)
Aristarchus of Samos undertook perhaps the first scientific based estimate for how far away our nearest cosmic neighbour, the Moon is. He used a method combining trigonometry (measuring the relative position angles of the Earth, Sun and Moon, when the Moon was at half-moon phase) with the size of the Earth’s shadow during lunar eclipses (which is where the Moon enters into the region of shadow cast by the Earth).
Aristarchus could determine his results for the relative distances of the Moon, and the Sun, from the Earth in terms of the number of Earth radii. Similar techniques were applied by later philosophers including Hipparcos and Ptolemy, and the Moon’s distance was estimated (by the later philosophers) as between 59 and 67 times the radius of the Earth. (The distance measured today by modern methods is just over 60 times the Earth’s radius.)
Estimates for the distances to the planets awaited the results of Copernicus, Brahe, Kepler and Newton in the 16th and 17th centuries, but again the distances could only be assessed in terms of relative scales, this time in terms of the mean distance between the Earth and the Sun. To determine actual measures in units which humans could understand, such as miles and/or kilometres, a method of determining the Earth’s size, and more importantly the Earth-Sun distance, was needed.
Edmund Halley (1656-1742), developing the work of James Gregory (1638-1675), showed that measuring the duration timings of transits of Venus provided such a method. The development and use in the 1960s of radar measurements of the distance from the Earth to the Moon, and the Earth to Venus distance enabled the scale of the solar system to be accurately established.
The distance from the Earth to the Sun is now known to be 149,597,870,700 metres (The distance varies slightly as the Earth’s orbit around the Sun is not perfectly circular). This is the definition of the Astronomical Unit (AU for short) and it is an important measure in Solar system work. However, having historically spent so much effort and time on determining the value of the AU, it is ironic that its true metre-equivalent value is now almost never used. The reason for this is that were it to be so, the numbers used in calculations and descriptions of solar system distances would be unwieldly. For example, Jupiter orbits the Sun at an average distance of 5.204 AU, which is 778,507,319,123 metres. As well as being cumbersome, this also doesn’t really convey meaning to humans who think in more modest sized units. (My walk into town is 2km, and that seems a reasonable distance. This is also a fine example of relativity though as it feels much longer in the rain…)
The distance to the stars is immensely further than the scale of the solar system and if we were to use the AU as a measuring ‘stick’, the closest star to the Sun (which is Proxima Centauri) would be said to be a little over 265,600 AUs away. So again, we will be in the realms of having to use large numeric values.
So, instead of the AU, the light-year (ly for short) is used as a standard measurement unit in stellar and galactic work. A light year is the distance travelled by a beam of light within a vacuum in the time of an Earth year (365.25 days, or 31,557,600 seconds). Whereas we see light being transmitted instantly, the electromagnetic radiation will see as light is actually propagated at 299,792,458 metres/second. So, one light year is the same as 9.46 x 10**15 metres. The distance to Proxima Centauri is 4.2 lights years.
An astronomical unit is the same distance as 8.32 light minutes. In other words, any light (or any other form of radiation) emanating from the solar photosphere would reach the Earth after 499 seconds. This does mean that if the Sun had just exploded, we wouldn’t know about it for at least 8 minutes 19 seconds. So you should have time to read the rest of this blog even if it has just done so.
Now, moving further away from the Earth we reach interstellar and galactic distances. Our Sun is one of at least one hundred thousand million other stars which all together comprise our ‘home’ galaxy, which we call the Milky Way. The Milky Way is a spiral galaxy and we are located within one of the spiral arms (called the Orion arm) about 27 thousand light years from the galactic centre. We orbit the galactic centre and it takes about 229 million years for us to complete one galactic revolution.
Many of the local stellar and interstellar distances are measured by high precision and accuracy parallax using astrometric satellites, especially the ESA’s observatories Hipparcos (Earth orbiting) and Gaia (positioned at the semi-stable gravitation point in the Solar system known as the Sun-Earth L(Lagrangian)2 point). These observatories use parallax to determine distances to relatively close (in the case of Gaia, up to 30,000 light years!) stellar objects.
In some galactic, and almost all intergalactic work, the Parsec (pc for short) is used as a measurement unit rather than the light year. A parsec (the word is derived from a parallax second of arc) is the distance at which the mean distance between the Earth and the Sun (i.e. the AU) subtends (‘makes’) one second of arc. In other units, it’s equivalent to 3.26 light years. So the Sun is 8.2Kpc from the galactic core of the Milky way
A galaxy similar to the Milky Way and a mere 2½ million light years away.
(Image used by couretsy of NASA/JPL)
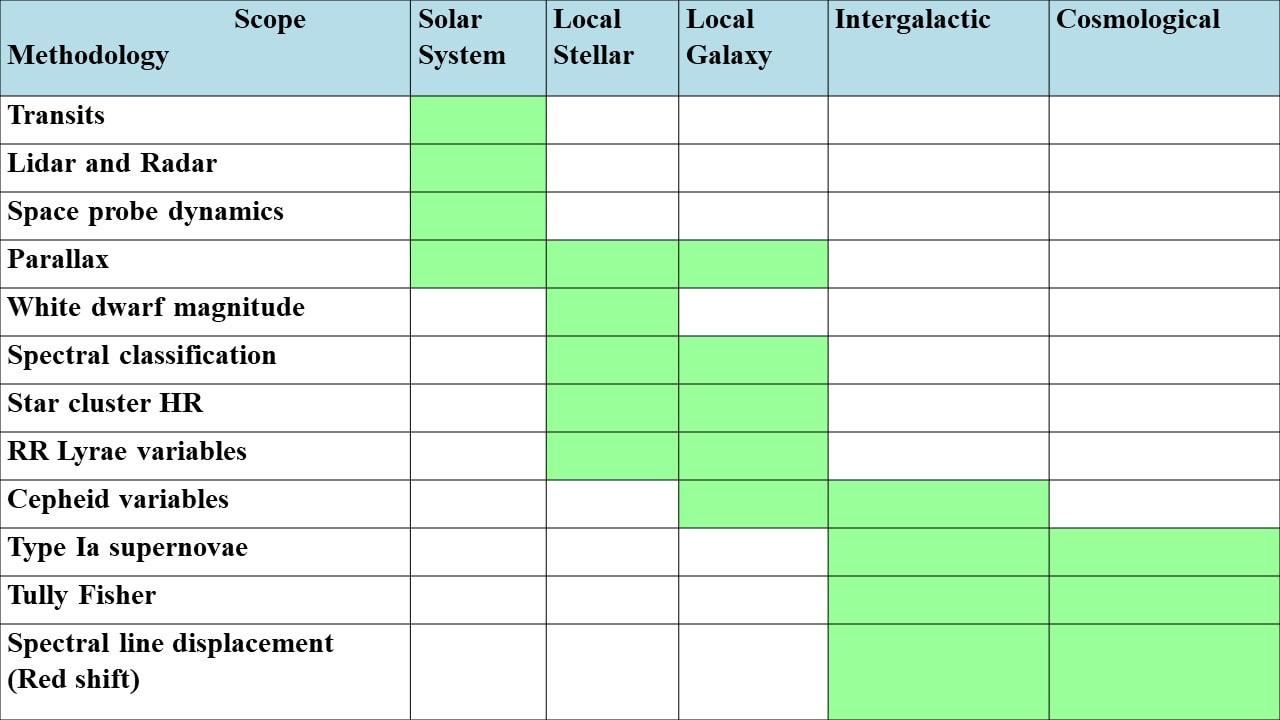
But how do we know about, and how can we measure, the vast distances to other stars and galaxies? We’ve talked a little bit about radar and parallax, but these can only be used for (astronomically speaking) very short distances. The following table shows a number of (the most widely used) techniques for measuring distances in astronomy and cosmology. Each of these methods would extend well beyond a blog post to describe in justice how they work, but the interested reader is encouraged to do their own research into these methods.
The size of the known Universe is integrally associated with the age of the Universe. Both are complex areas of study (cosmology) and we will here just note that the age of the Universe is currently estimated to be around 13.8 billion years. The most remote objects we have detected (quasars) are at around 13.1 billion light years distant from us. As we are not specialists in cosmology, we will defer what ‘age’ and ‘distance’ mean in a cosmological context, and how they are related, to another time. (Please excuse the rather obvious pun!)
https://www.nasa.gov/content/hubbles-double-take
(In ascending level of technical complexity)
The Sun – shining light on the Solar system. Neil Taylor. 2017
http://www.observatoiresolaire.eu/solar-physics.html
Astrophysics Processes. Hale Bradt. 2008
http://www.cambridge.org/gb/academic/subjects/physics/astrophysics/astrophysics-processes-physics-astronomical-phenomena?format=PB#zwL37giFPr7j95zo.97
Deconstructing Cosmology. Robert Sanders. 2016
http://www.cambridge.org/gb/academic/subjects/physics/cosmology-relativity-and-gravitation/deconstructing-cosmology?format=HB#WPh0IPZexb4upZmx.97
We will return to our series on the Sun in next month’s blog. Having looked at the core of the Sun, we will next look at the Sun’s atmosphere and how events such as flares and the solar wind could spell trouble for life on Earth. We will begin our review by considering atmospheres in general, and the factors involved in any star or planet retaining an atmosphere.
We will see why we have virtually no molecular or atomic hydrogen or helium within the Earth’s atmosphere, despite these elements accounting for more than 98% of all known baryonic matter in the Universe.
Next month’s blog will be issued on Saturday 27th January 2018